(1) Samir Chitnavis, School of Biological and Behavioural Sciences, Queen Mary University of London, Mile End, London E1 4NS, UK & Digital Environment Research Institute, Queen Mary University of London, Empire House, Whitechapel E1 1HH, UK;
(2) Thomas J. Haworth, Astronomy Unit, Queen Mary University of London, Mile End Road, London E1 4NS, UK;
(3) Edward Gillen, Astronomy Unit, Queen Mary University of London, Mile End Road, London E1 4NS, UK;
(4) Conrad W. Mullineaux, School of Biological and Behavioural Sciences, Queen Mary University of London, Mile End, London E1 4NS, UK;
(5) Christopher D. P. Duffy, School of Biological and Behavioural Sciences, Queen Mary University of London, Mile End, London E1 4NS, UK & Digital Environment Research Institute, Queen Mary University of London, Empire House, Whitechapel E1 1HH, UK (Email: c.duffy@qmul.ac.uk).
Table of Links
- Abstract and Introduction
- 2 Methodology
- 2.1 Local spectral irradiance as a function of stellar temperature
- 2.2 Thermodynamic model of an oxygenic light-harvesting system
- 2.3 Lattice model of an oxygenic light-harvesting system
- 3 Results
- 3.1 Orbital distances and incident spectral fluxes
- 3.2 Thermodynamic antenna model: Increasing antenna size in limited PAR
- 3.3 Lattice antenna model: Increasing the size of a “flat” antenna in limited PAR
- 3.4 Lattice antenna model: Improving antenna efficiency with an energetic ’funnel’
- 4 Discussion
- Acknowledgements, Author Contribution Statement, Authors disclosure statement and References
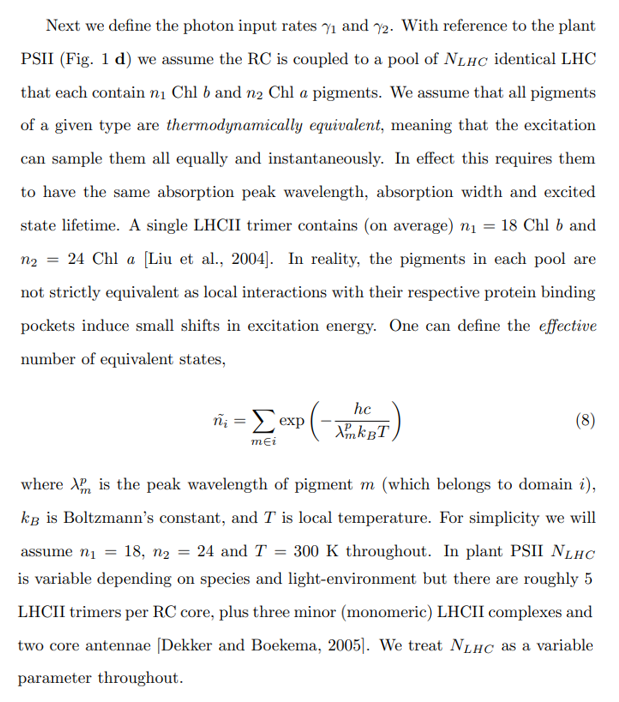
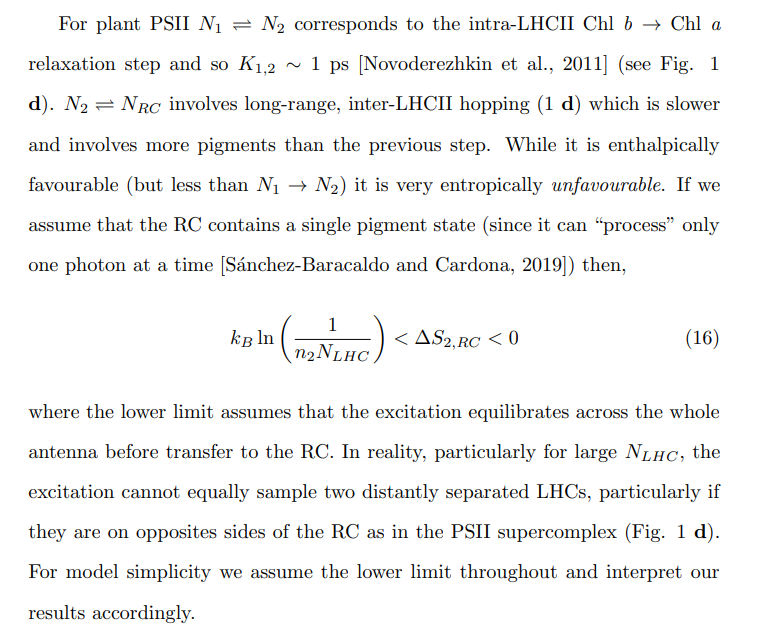
2.2 Thermodynamic model of an oxygenic light-harvesting system
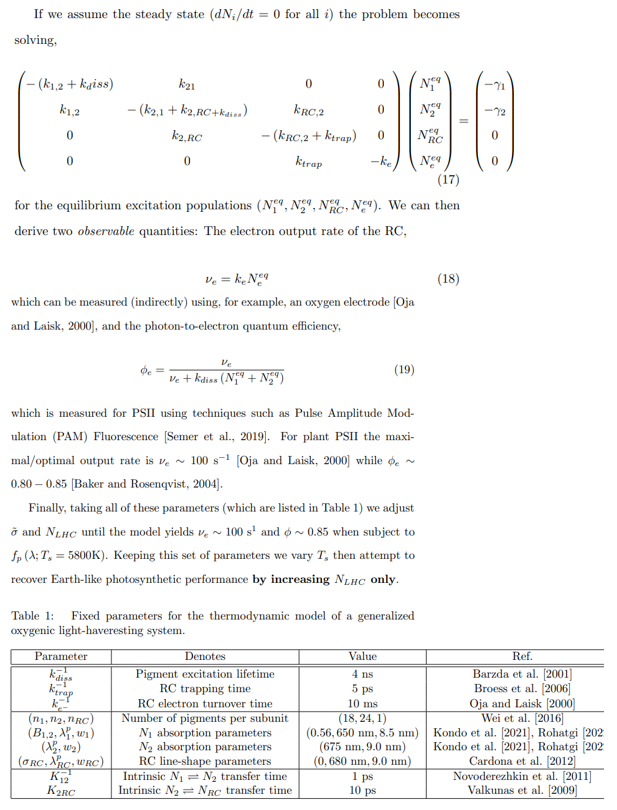
Our first light-harvesting model is general, explicitly thermodynamic and based loosely on PSII of vascular plants. However, the exercise is not to precisely simulate the light-harvesting dynamics of plants but to compare the performance of a qualitative model in different spectral irradiances. A schematic of the model is shown in Fig. 2 a. We assume that the antenna is composed of two energetically distinct domains which in plants are the interpenetrating Chl a and b pools. At time, t, these two pools contain N1 (t) and N2 (t) excitations respectively. Energy is transferred sequentially from N1 to N2 to the RC, whose excitation occupation is denoted NRC (t). The RC then traps this excitation by converting it into a charge separated state, which we assume is irreversible. The trap states, Ne− (t), represents the meta-stable charge-separated state that eventually reduces the (quinone) electron acceptor. The equations of motion for this system are,


The transfer rates therefore satisfy the detailed balance condition,

This paper is available on arxiv under CC 4.0 license.